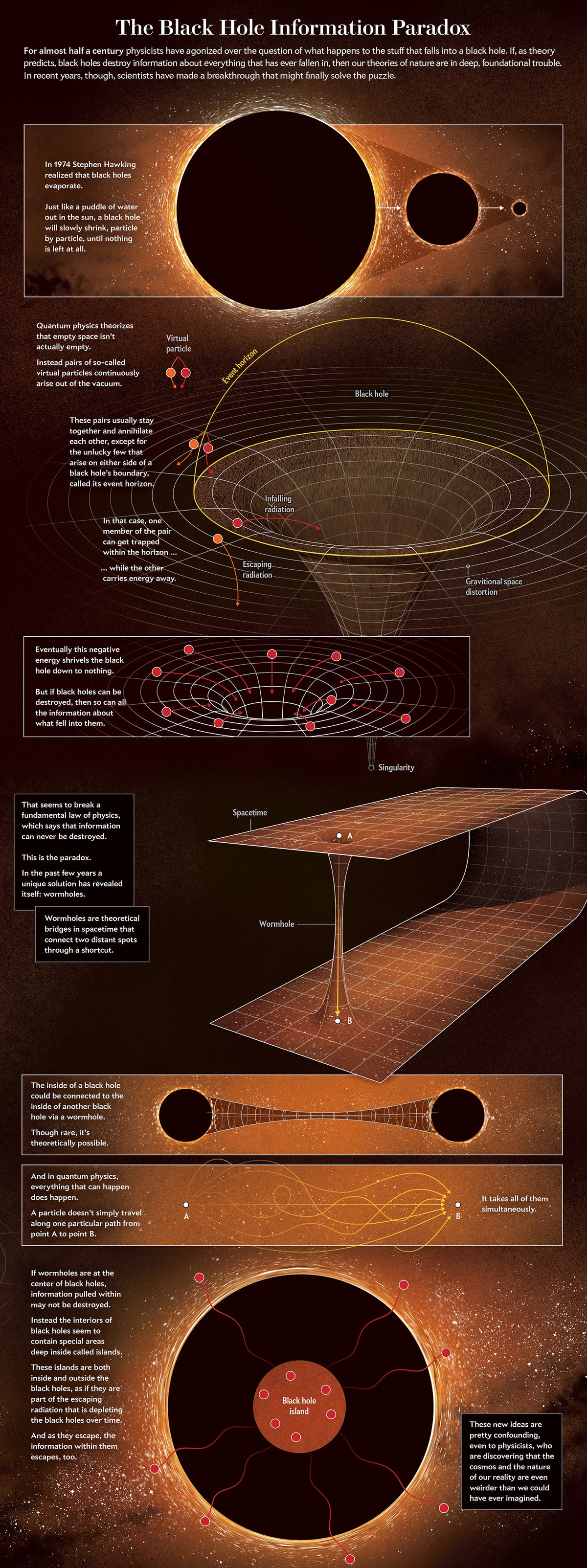
Theoretical physics has been in crisis mode ever since 1974, when Stephen Hawking argued that black holes destroy information. Hawking showed that a black hole can evaporate, gradually transforming itself and anything it consumes into a featureless cloud of radiation. During the process, information about what fell into the black hole is apparently lost, violating a sacred principle of physics.
This remained an open problem for almost 50 years, but the pieces started falling into place in 2019 through research that I was involved in. The resolution is based on a new understanding of spacetime and how it can be rewired through quantum entanglement, which leads to the idea that part of the inside of a black hole, the so-called island, is secretly on the outside.
To understand how we arrived at these new ideas, we must begin with the inescapable nature of black holes.
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
A One-Way Street
Nothing seems more hopeless than trying to get out of a black hole—in fact, this impossibility is what defines black holes. They are formed when enough matter is confined within a small enough region that spacetime collapses in on itself in a violent feedback loop of squeezing and stretching that fuels more squeezing and stretching. These tidal forces run to infinity in finite time, marking the abrupt end of an entire region of spacetime at the so-called black hole singularity—the place where time stops and space ceases to make sense.
There is a fine line within the collapsing region that divides the area where escape is possible from the point of no return. This line is called the event horizon. It is the outermost point from which light barely avoids falling into the singularity. Unless a thing travels faster than light—a physical impossibility—it cannot escape from behind the event horizon; it is irretrievably stuck inside the black hole.
The one-way nature of this boundary is not immediately problematic. In fact, it is a robust prediction of the general theory of relativity. The danger starts when this theory interacts with the wild world of quantum mechanics.
Something Out of Nothing
Quantum theory redeems black holes from being the greedy monsters they are made out to be. Every calorie of energy they consume they eventually give back in the form of Hawking radiation—energy squeezed out of the vacuum near the event horizon.
The idea of getting something out of nothing may sound absurd, but absurdity is not the worst allegation made against quantum mechanics. The emptiness of the vacuum in quantum theory belies a sea of particles—photons, electrons, gravitons, and more—that conspire to make empty space feel empty. These particles come in carefully arranged pairs, acting hand in hand as the glue that holds spacetime together.
Particle pairs that straddle the event horizon of a black hole, however, become forever separated from each other. The newly divorced particles peel away from the horizon in opposite directions, with one member crashing into the singularity and the other escaping the black hole's gravitational pull in the form of Hawking radiation. This process is draining for the black hole, causing it to get lighter and smaller as it emits energy in the form of the outgoing particles. Because of the law that energy must be conserved, the particles trapped inside must then carry negative energy to account for the decrease in the total energy of the black hole.
From the outside, the black hole appears to be burning away (although it happens so slowly, you can't see it happening in real life). When you burn a book, the words on its pages imprint themselves on the pattern of the emanating light and the remaining ashes. This information is thus preserved, at least in principle. If an evaporating black hole were a normal system like the burning book, then the information about what falls into it would be encoded into the emerging Hawking radiation. Unfortunately, this is complicated by the quantum-mechanical relation among the particles across the horizon.
Credit: Matthew Twombly
Einstein's Enemy
The issue begins with the end of the pairing of the two particles straddling the event horizon. Despite being separated, they maintain a quantum union that transcends space and time—they are connected by entanglement. Rejected as an absurdity by the physicists who predicted it, quantum entanglement is perhaps one of the weirdest aspects of our universe and arguably one of its most essential. The concept was first concocted by Albert Einstein, Boris Podolsky and Nathan Rosen as a rebuttal against what was then the nascent theory of quantum mechanics. They cited entanglement as a reason the theory must be incomplete—“spooky” is how Einstein famously described the phenomenon.
For a simple example of entanglement, consider two coins in a superposition—the quantum phenomenon of being in multiple states until a measurement is made—of both coins being either heads or tails. The coins aren't facing heads and tails at the same time—that's physically impossible—but the superposition signifies that the chance of observing the pair of coins in either orientation, both heads or both tails, is a probability of one half. There is no chance of ever finding the coins in opposite orientations. The two coins are entangled; the measurement result of one predicts the result of the other with complete certainty. Either coin by itself is completely random, devoid of information, but the randomness of the pair is perfectly correlated.
The scientists were troubled by how the two coins appeared to influence each other without having to come into physical contact. The coins could be in separate galaxies while still maintaining the same amount of entanglement between them. Einstein was unnerved by the apparent “spooky action at a distance” linking the results of the two separate random measurements.
The irony is that Einstein himself is in a superposition of being both wrong and right. He was right to recognize the importance of entanglement in distinguishing quantum mechanics from classical physics. What he got wrong can be summed up with the truism “correlation does not imply causation.” Although the fates of the particles are inextricably correlated, the measurement outcome of one does not cause the outcome of the other. It turns out that quantum mechanics simply allows for a new, higher degree of correlation than we are used to.
Information Lost
Because Hawking radiation is composed of one half of a collection of entangled pairs, it emerges from the black hole in a completely random state—if the particles were coins, they would be observed to be heads or tails with equal probability. Hence, we cannot infer anything useful about the contents of the black hole from the random measurements of the radiation. This means that an evaporating black hole is basically a glorified information shredder, except unlike the mechanical kind, it does a thorough job.
We can measure the lack of information—or the randomness—in the Hawking radiation by thinking about the amount of entanglement between the radiation and the black hole. This is because one member of an entangled pair is always random, and the outside members are all that remains by the end of the evaporation. The calculation of randomness goes by many names, including entanglement entropy, and it grows with every emerging Hawking particle, plateauing at a large value once the black hole has completely disappeared.
This pattern differs from what happens when information is preserved, as in the example of a burning book. In such a case, the entropy may rise initially, but it has to peak and then fall to zero by the end of the process. The intuition behind this rule is clear when you think about a standard deck of cards: suppose you are dealt cards from a 52-card deck, one by one, facing down. The entropy of the cards in your possession is simply a measure of your ignorance of what's on the other side of the cards—specifically, the number of possibilities of what they could be. If you have been dealt just one card, the entropy is 52 because there are 52 possibilities. But as you are dealt more, the entropy rises, peaking at 500 trillion for 26 cards, which could be any of 500 trillion different combinations. After this, though, the possible mixes of cards, and thus the entropy, go back down, reaching 52 again when you have 51 cards. Once you have all the cards, you are certain of exactly what you have—the entire deck—and the entropy is zero. This rising and falling pattern of entropy, known as the Page curve, applies to all normal quantum-mechanical systems. The time at which the entropy peaks and then starts to decrease is the Page time.
The destruction of information inside black holes spells disaster for physics because the laws of quantum mechanics stipulate that information cannot be obliterated. This is the famous information paradox—the fact that a sprinkling of quantum mechanics onto the description of black holes leads to a seemingly insurmountable inconsistency. Physicists knew we needed a more complete understanding of quantum-gravitational physics to generate the Page curve for the Hawking radiation. Unsurprisingly, this task proved difficult.
An Eventful Horizon
Part of the challenge was that no minor tweaking of the evaporation process was sufficient to generate the Page curve and send the entropy back down to zero. What we needed was a drastic reimagining of the structure of a black hole.
In a paper I published in 2013 with Donald Marolf, the late Joseph Polchinski, and Jamie Sully (known collectively as AMPS), we tried out several ways to modify the picture of evaporating black holes using a series of gedankenexperiments—the German term for the kind of thought experiments Einstein popularized. Through our trials we concluded that to save the sanctity of information, one of two things had to give: either physics must be nonlocal—allowing for information to instantaneously disappear from the interior and appear outside the event horizon—or a new process must kick in at the Page time. To preclude the increase of entropy, this process would have to break the entanglement between the particle pairs across the event horizon. The former option—making physics nonlocal—was too radical, so we decided to go with the latter.
This modification helps to preserve information, but it poses another paradox. Recall that the entanglement across the horizon was a result of having empty space there—the way the vacuum is maintained by a sea of entangled pairs of particles. The entanglement is key; breaking it comes at the cost of creating a wall of extremely high-energy particles, which our group named the firewall. Having such a firewall at the horizon would forbid anything from entering the black hole. Instead infalling matter would be vaporized on contact. The black hole at the Page time would suddenly lose its interior, and spacetime would come to an end, not at the singularity deep inside the black hole but right there at the event horizon. This conclusion is known as the firewall paradox, a catch-22 that meant any solution to the information paradox must come at the cost of destroying what we know about black holes. If ever there were a quagmire, this would be it.
Fluctuating Wormholes
Eventually my colleagues and I realized that both the information paradox and the newer firewall paradox arose because our attempts to meld quantum mechanics and black hole physics were too timid. It wasn't enough to apply quantum mechanics to only the matter present in black holes—we had to devise a quantum treatment of the black hole spacetime as well. Although quantum effects on spacetime are usually very small, they could be enhanced by the large entanglement produced by the evaporation. Such an effect may be subtle, but its implications would be huge.
To consider the quantum nature of spacetime, we relied on a technique designed by Richard Feynman called the path integral of quantum mechanics. The idea is based on the weird truth that, according to quantum theory, particles don't simply travel along a single path from point A to point B—they travel along all the different paths connecting the two points. The path integral is a way of describing a particle's travels in terms of a quantum superposition of all possible routes. Similarly, a quantum spacetime can be in a superposition of different complicated shapes evolving in different ways. For instance, if we start and end with two regular black holes, the quantum spacetime within them has a nonzero probability of creating a short-lived wormhole that temporarily bridges their interiors.
Usually the probability of this happening is vanishingly slim. When we carry out the path integral in the presence of the Hawking radiation of multiple black holes, however, the large entanglement between the Hawking radiation and the black hole interiors amplifies the likelihood of such wormholes. This realization came to me through work I did in 2019 with Thomas Hartman, Juan Maldacena, Edgar Shaghoulian and Amirhossein Tajdini, and it was also the result of an independent collaboration by Geoffrey Penington, Stephen Shenker, Douglas Stanford and Zhenbin Yang.
Islands beyond the Horizon
Why does it matter if some black holes are connected by wormholes? It turns out that they modify the answer of how much entanglement entropy there is between the black hole and its Hawking radiation. The key is to measure this entanglement entropy in the presence of multiple copies of the system. This is known as the replica trick.
The relevant physical effect of these temporary wormholes is to swap out the interiors among the different black holes. This happens literally: what was in one black hole gets shoved into one of the other copies far away, and the original black hole assumes a new spacetime interior from a different one. The swapped region of the black hole interior is called the island, and it encompasses almost the entire interior up to the event horizon.
The swapping is exactly what the doctor ordered! Focusing on one of the black holes and its Hawking radiation, the swapped-out island takes with it all the partner particles that are entangled with the outgoing Hawking radiation, and hence, technically, there is no entanglement between the black hole and its radiation.
Including this potential effect of wormholes produces a new formula for the entanglement entropy of the radiation when applied to a single copy of the system. Instead of Hawking's original calculation, which simply counts the number of Hawking particles outside a black hole, the new formula curiously treats the island as if it were outside and a part of the exterior Hawking radiation. Therefore, the entanglement between the island and the exterior should not be counted toward the entropy. Instead the entropy that it predicts comes almost entirely from the probability of the swap actually occurring, which is equal to the area of the boundary of the island—roughly the area of the event horizon—divided by Newton's gravitational constant. As the black hole shrinks, this contribution to the entropy decreases. This is the island formula for the entanglement entropy of the Hawking radiation.
The final step in computing the entropy is to take the minimum between the island formula and Hawking's original calculation. This gives us the Page curve that we've been after. Initially we calculate the entanglement entropy of the radiation with Hawking's original formula because the answer starts off smaller than the area of the event horizon of the black hole. But as the black hole evaporates, the area shrinks, and the new formula takes the baton as the true representative of the radiation's entanglement entropy.
What is remarkable about this result is that it solves two paradoxes with one formula. It appears to address the firewall paradox by supporting the option of nonlocality that my AMPS group originally dismissed. Instead of breaking the entanglement at the horizon, we are instructed to treat the inside—the island—as part of the outside. The island itself becomes nonlocally mapped to the outside. And the formula solves the information paradox by revealing how black holes produce the Page curve and preserve information.
Let's take a step back and think about how we got here. The origins of the information paradox can be traced back to the incompatibility between the sequestering of information by the event horizon and the quantum-mechanical requirement of information flow outside the black hole. Naive resolutions of this tension lead to drastic modifications of the structure of black holes; however, subtle yet dramatic effects from fluctuating wormholes change everything. What emerges is a self-consistent picture that lets a black hole retain its regular structure as predicted by general relativity, albeit with the presence of an implicit though powerful nonlocality. This nonlocality is screaming that we should consider a portion of the black hole's interior—the island—as part of the exterior, as a single unit with the outside radiation. Thus, information can escape a black hole not by surmounting the insurmountable event horizon but by simply falling deeper into the island.
Despite the excitement of this breakthrough, we have only begun to explore the implications of spacetime wormholes and the island formula. Curiously, while they ensure that the island is mapped onto the radiation, they do not generate a definite prediction for specific measurements of the Hawking radiation. What they do teach us, however, is that wormholes are the missing ingredient in Hawking's original estimation of the randomness in the radiation and that gravity is in fact smart enough to comply with quantum mechanics.
Through these wormholes, gravity harnesses the power of entanglement to achieve nonlocality, which is just as unnerving to us as the entanglement that originally spooked Einstein. We must admit that, at some level, Einstein was right after all.
WATCH THIS NEXT

